The CPA Approach – Macmillan International Curriculum

Welcome to our blog on the CPA approach, written by primary maths consultant, Andrew Jeffrey. At the end of this post, you will find a FREE exemplar lesson plan, which we hope you will find useful.

Introduction

The term ‘CPA’, which stands for ‘Concrete, Pictorial, and Abstract’, has come to prominence amongst teachers in recent years, with the increasing popularity of “Singapore-style” teaching methods. It refers to the theory of introducing potentially tricky abstract mathematical ideas by first using quantities of physical objects, then by showing images or representations of those objects, and finally by using symbols to represent them.

Yet this approach did not originate with the Singaporeans. In the 1960s American educationalist Jerome Bruner introduced what he referred to as the ‘enactive, iconic and symbolic’ phases of learning. Enactive is about physically doing, iconic is about representing in recognisable form, including language, while the symbolic phase involves using non-motivated symbols, i.e. symbols that do not resemble that which they represent. 4 and 5 are a good example; it is impossible to know just from looking at the shapes of the numerals which is the larger number.

Practical Example

For early addition and subtraction, this might involve two sets of objects, for example pencils. Here we have a set of six, and one more. We can physically combine them and count the total. Next we can draw this out in a range of ways – here are just three: tens frames, tally marks and Numicon shapes. Finally, we learn to represent this using the abstract symbols we refer to as numerals.

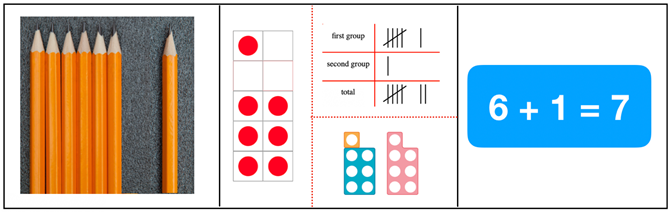
Sequencing

This is often misunderstood. Bruner did not talk about replacing enactive with iconic, or iconic with symbolic. He used the phrase ‘move between representations’ to infer a flexibility to switch back and forth rather than a one-way journey from objects to symbols. The idea is that children should feel free to use equipment to explore mathematical ideas whatever stage they are at, or to return to imagery even once concepts are understood.

For example, when thinking about division, a teacher might ask children to find the value of 20 ÷ 5. (abstract). If children were unsure how to proceed, she might choose to demonstrate with a number line (pictorial) as shown here:

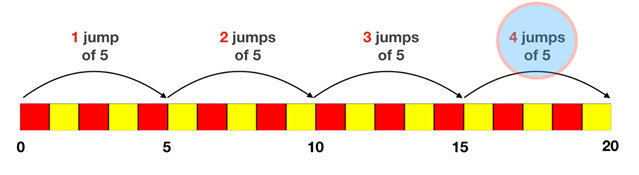
If required, the teacher also has the option to go further, perhaps by drawing groups of five dots and exploring how many are needed to make a total of 20 dots. This is still pictorial of course, but if required children could return to the concrete phase, perhaps with Rods or Numicon:

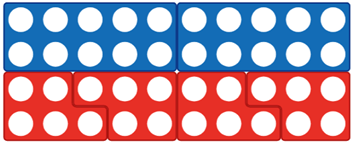
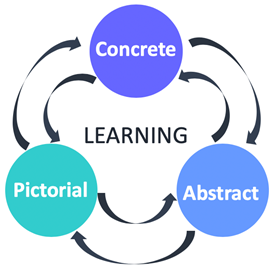
Thus we can see that learning through manipulatives is not a linear process. It can perhaps best be thought of in this way:

Selecting Manipulatives

It is very important when planning learning episodes, that we give considerable thought to which resources are the most appropriate. (Boonen, van Wesel, Jolles & van der Schoot, (2014) ran a study suggesting that students who make use of appropriate concrete visual resources in a CPA approach, have a 6-fold advantage in solving mathematical word problems over those who do not. But they also found that using the wrong ones can actually reduce the chance of students reaching a correct solution. For example, when thinking about place value and the number 132, the image above the line in the following diagram is far more appropriate and will lead to fewer misconceptions that the one below it, because it attends to the structure of the number and the different meanings of each column:

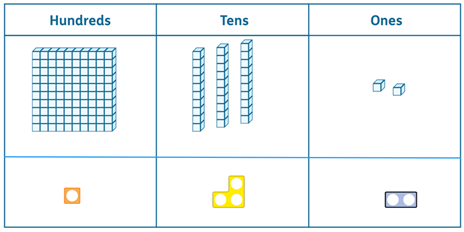
Therefore, it is very important that we consider both the structure and the concept we wish children to grasp before selecting the best resource for the job.

View and download your free exemplar lesson plan here!

Introduction

Practical Example

Sequencing

Selecting Manipulatives

FURTHER READING